A Tonnetz in Practice

Stephanie Leotsakos
Rutgers University
December 2020

1 The Tonnetz and its many iterations have traditionally been used in the context of post-graduate music theory studies, most prominently in the areas of Neo-Riemannian transformational theory and of the geometry of music. It is not commonly used in the undergraduate theory core curriculum or in everyday music lessons, whether instrumental or compositional. If it is, it is only referenced tangentially. What if a Tonnetz was used to teach core music theory concepts from the start, beginning at the fundamental level? The use of a supplemental visual aid would help students grasp abstract theoretical concepts more easily and learn to re-construct and de-construct fundamental aspects of tonal harmony with greater command themselves. By the time students complete AP Music Theory in high school, or music theory levels 1 and 2 in undergraduate programs, this content and these skills are what students are expected to have mastered. Yet, there remains a wide spectrum of student understanding and proficiency in music theory classrooms, making it more difficult for educators to address the needs of all. Sometimes struggling students are left to their own devices or must seek out extra help or tutoring, and others, though they may perform alright in the class, might not go on to really use that knowledge in practice outside of the classroom. Music theory should not always be taught in isolation separate from practice, but if it is going to be, it would be best to make it more obviously practical. Using a Tonnetz as a constructivist tool and referential visual aid would benefit student understanding across the spectrum within music theory classrooms and could make for a practical or pedagogical tool outside the classroom.

2 Though the average musician, even at the undergraduate level, has likely never heard of a Tonnetz, those who have might recall the most traditionally known 19th-century Neo-Riemannian Tonnetz, a “note-based” Tonnetz built using equilateral triangles which, according to Professor Dmitri Tymoczko, represents notes through its points and chords through the extended shapes created by those points.[1] There are many other graphical representations of Tonnetze including the chord-based “chicken-wire” Tonnetz rendered by Jack Douthett and Peter Steinbach in 1998, which Tymoczko explains represents entire sonorities at its graphical points and illustrates efficient voice leading via short distances in space (Figure 1).

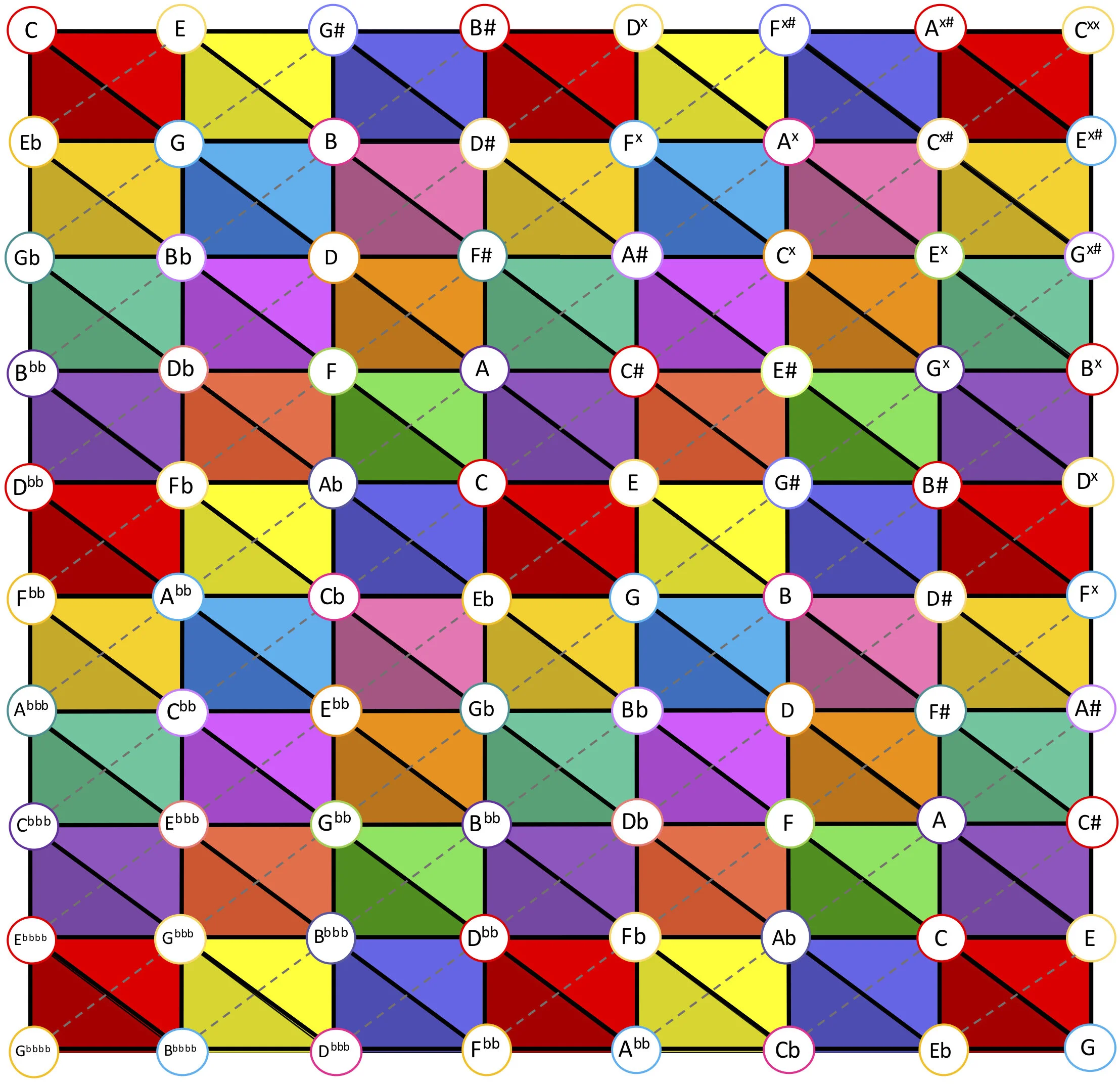
3 For the purposes of teaching theory topics beginning in level one theory classes however, I propose using a note-based Tonnetz, one which illustrates intervallic distances of major/minor thirds and perfect fifths via “3-4-5” right-triangles, not equilateral, and uses color to further identify major and minor triads and enharmony (see Figures 2.1 and 2.2). One can just as easily map efficient voice leading in short spatial distances with this Tonnetz as well. The Tonnetz is an integrative and interactive tool well-suited to helping students approach music theory in a logically constructive and visually mechanical way, without the need of learning algorithmic transformations like those in Neo-Riemannian theory. Figures 2.1 and 2.2 below illustrate two renditions of the type of Tonnetz I propose putting into practice.

4 The Tonnetze in figures 2.1 and 2.2 are primarily visualization tools, ones which bridge mechanical learning with musical thinking, grounded in an easily generated and illustrated logic. The music theory concepts which can be taught are not dependent on performance ability or even on great staff-notation literacy (though it can help develop it). A Tonnetz is a great tool to start with, as it can be built piece-by-piece in an intuitive way, at any learning pace necessary and at any age, with just a few foundational elements. Music theory level one and two topics the Tonnetz would aid in learning include (in no particular order): staff and clef literacy, enharmony and spelling, interval learning (m2, M2, m3, M3, P5, etc), chromaticism, Major/minor triads (including parallel and relative) and their functional relationships, augmented and diminished triads, circle of fifths, 7th (9th+) chords, inversions, voice leading, cadential and other harmonic patterns, roman numerals, transposition, sequences, pitch centricity, and more.

5 With my Tonnetz, I do not propose eliminating the use of the piano from the curriculum, only to supplement it, and perhaps even provide a means of navigating the piano better by visualizing harmony in a unified way. The goal is for music theory to ultimately be applicable to musicians across all instruments and to enhance “musical thinking.” To accomplish this, a tool for learning that does not favor any single instrument in particular is very useful for keeping everyone on the same page in many different settings. Understanding the inherent geometry that tertian pitch relationships form is an efficient way to construct and navigate harmonic, sequential, and transpositional elements in music in a way that is less arbitrary than when learned directly at the piano or using only the staff. Key signatures and intervallic distances on the staff are supposed to give us much of this information, but many other aspects like inversion, transposition, or voice leading still expect the student to imagine this information with their mind’s eye, usually visualizing an imagined staff or piano. It has been my experience that the Tonnetz provides an intermediary tool that helps transference of information from the initial learning stage into consistent practice, whether notated or performed.

6 There is a longstanding tradition of using analogues and references from the visual-art world in the music-world because often music seems esoteric and difficult to describe. There has also been a fascination with understanding phenomena like synesthesia, where sound (musical pitches, chords, key areas, etc.) is “seen” or described as color or texture– even influencing musical composition, as was the case with composers Scriabin, Messiaen, Sibelius, Ligeti, Liszt, and others. Aristotle is credited with having been the first to observe a relationship between music and color, albeit philosophically, and since then, researchers, musicians, and theorists across all academic disciplines have philosophized, theorized, and experimented in pursuit of a better understanding of how sound and ‘music’ functionally relates physically, mathematically, emotionally, psychologically, and visually to human expression in music and art. The literal study of color and sound, of tone-color or pitch-color, separate from that of timbre, has been varied and inconsistent. However, we still see color used as a teaching tool in method books for children and in musical toys, though also inconsistent and seemingly assigned arbitrarily to different musical notes.

7 My choice of primary colors mainly aimed to outline the first triad I would teach at the piano, C-Major, so that a logical progression of secondary colors might be “discoverable” as a musical “color-wheel,” since most children by age four or five have already been introduced to color vocabulary and can identify colors with ease, even prior to learning the alphabet and understanding the function of “letters.” Because I first began developing this method and Tonnetz with a younger age group, the seven distinct colors assigned to the first seven notes of the “musical alphabet” were a result of my experience with a younger age group.  Though not entirely necessary at a more advanced level, I do advocate its use, as I find it helps to view and assimilate harmonic patterns much more quickly and with aesthetic enjoyment. Figure 3 is the initial seven-note color-wheel I created (Figure 3).

8 Following suit, tertiary color mixtures indicate sharp or flat pitches of a tempered system, i.e. chromaticism (Figure 4). I like to identify the color-mixture of these notes based on their name, though the color itself remains equivalent just as the enharmonic pitch would on the piano. For example, C# would be identified as “red-orange” while Db would be “orange-red,” though their color is exactly the same. Similarly, this applies to the color of enharmonic triads in my Tonnetz as well, where the color of a C#-major triad is the the same Db-major’s, for example, making progressive harmonic travel much easier to follow on a Tonnetz that is based on harmonic repetition and pattern.

10 To begin to build my two-dimensional Tonnetz, each triad is constructed with two sides of differing lengths, Major and minor thirds of lengths 4 and 3 semitones, linked at a 90-degree angle, and resulting in a hypotenuse representative of the perfect 5th interval (in reality, 7 semitones in length but projected as a Perfect 5th in a two dimensional plane). Via the same logic, an inverse construction results in the parallel minor triad and is represented using a darker shade of its parallel’s color (Figures 6.1 and 6.2):

12 One can then easily glean a graphical short hand for inversions for any triad, seventh, ninth, or larger chords
(Figure 8):

13 Using this orientation, where major-third intervals are horizontal and minor-third intervals are vertical, augmented and diminished chords become easily identifiable, though they do not retain a triangular shape as the major and minor triads do. This is extremely fitting however, as most often diminished or augmented chords function transitorily in music, to get from one harmonic destination to another. Assuming a path of least resistance, augmented and diminished chords propel harmony quickly in other directions as they would across the Tonnetz chart.

14 Seventh chords result as a natural extension of triads simply by adding on a major or minor third to the triad’s original configuration. Such an extension makes seventh chords take on slightly different shapes, making them memorable and logical in construction for the student (Figure 9). For example, a half-diminished 7th chord is easily identifiable as the inverse of a dominant 7th chord. Furthermore, the context of their harmonic functions start to become apparent when progressions are mapped in action on the Tonnetz.

15 With the Tonnetz, we can also identify perfect 5th relationships at an easy glance, and thus teach the circle of fifths in an integrated way (Figure 10). It is made easier to identify by then warping unto itself in circular formation as we see in [Figure 11], outlining the initial structure of what would eventually be a Tonnetz-torus (Figure 12).

16 Additionally, Roman numerals, harmonic relationships, cadential patterns, chromaticism, and clef transposition can be taught in any major or minor key (Figures 13 and 14).

17 Eventually, the Tonnetz can also be used as a tool for harmonic analysis and observing harmonic directionality in music at higher levels. It can aid discovery and visualization of larger patterns and symmetries in musical forms (Figure 15.1 -15.6 ).

18 Having overviewed various ways in which a colored, ‘Pythagorean’ Tonnetz can be versatile, we glimpse how our harmonic system is more symmetrical and beautifully rounded than students often understand when regurgitating mechanics learned in music theory class. Though the Tonnetz visually aids the learning of those mechanics and grounds students in the arithmetic and grammar of harmony, it does so while situating them more clearly into a larger ‘functional’ system—one that is demystified and approachable to students of any background or age. It can be practical and consequently boost morale if helpful to even a small percentage of students, especially those who are struggling, though I personally believe it would service the majority if skillfully used in instruction. So much information can be visualized in a small space such that often-used words like ‘distance,’ ‘direction,’ ‘movement,’ ‘sequence,’ and ‘progression,’ take on new meaning. Music’s minute details can be scaled up and larger forms and structures can be scaled down in a geographical system that expands or contracts according to need. Pattern discovery leads to pattern assimilation, reproduction, and synthesis in practice—a helpful tool for composition, analysis, and improvisation. Should a more advanced student become interested, a dive into studying transformational theory or the geometry of music becomes less of a leap, as it is already preceded by familiarity with the Tonnetz and accustomed abstract thinking. In the realm of music theory, fluency and performance skill should supersede being a mechanical exercise, just as “thinking in music” should tap into a more abstract, yet integrated, level of understanding. I believe the Tonnetz has been an underrated tool in the pursuit of this goal, and my hope is to see it become more widely used in music theory curriculums.

FOOTNOTES

[1] Tymoczko Dmitri, “The Generalized Tonnetz,” Journal of Music Theory vol 56. no 1., (Spring 2012): 1-2.

BIBLIOGRAPHY

Cohn, Richard, “Introduction to Neo-Riemannian Theory: A Survey and a Historical

Perspective.” Journal of Music Theory, Vol. 42, No. 2., (Autumn 1998): 167 – 180.

Cohn, Richard, “Neo-Riemannian Operations, Parsimonious Trichords, and Their ‘Tonnetz’

Representations.” Journal of Music Theory, vol. 41, no.1., (1997): 1–66.

https://doi.org/10.2307/843761. 

Engebretsen, Nora, and Per Broman. “Transformational Theory in the Undergraduate

Curriculum: A Case for Teaching the Neo-Riemannian Approach.” Journal of Music

Theory Pedagogy, vol.21., (January 2007). http://search.proquest.com/docview/1416837/.

Pierce, Arthur, “Color and Music.” The American Mercury 23 (June 1931).

http://search.proquest.com/docview/1296004045/.

Rings, Steven. Tonality and Transformation New York: Oxford University Press, 2011.

Santa, Matthew, “Teaching Non-Functional Tonality: A Part-Writing Approach.” Journal

of Music Theory Pedagogy 23 (January 2009): 79–100. http://search.proquest.com/docview/1283952505/.

Tymoczko, Dmitri. A Geometry of Music: Harmony and Counterpoint in the Extended

Common Practice. New York: Oxford University Press, Incorporated, 2011.

Tymoczko, Dmitri, “The Generalized Tonnetz.” Journal of Music Theory, vol. 56, no.1., (Spring

2012): 1- 52. https://doi.org/10.1215/00222909-1546958.